This will be a talk for the CUNY Set Theory Seminar, March 6, 2015.
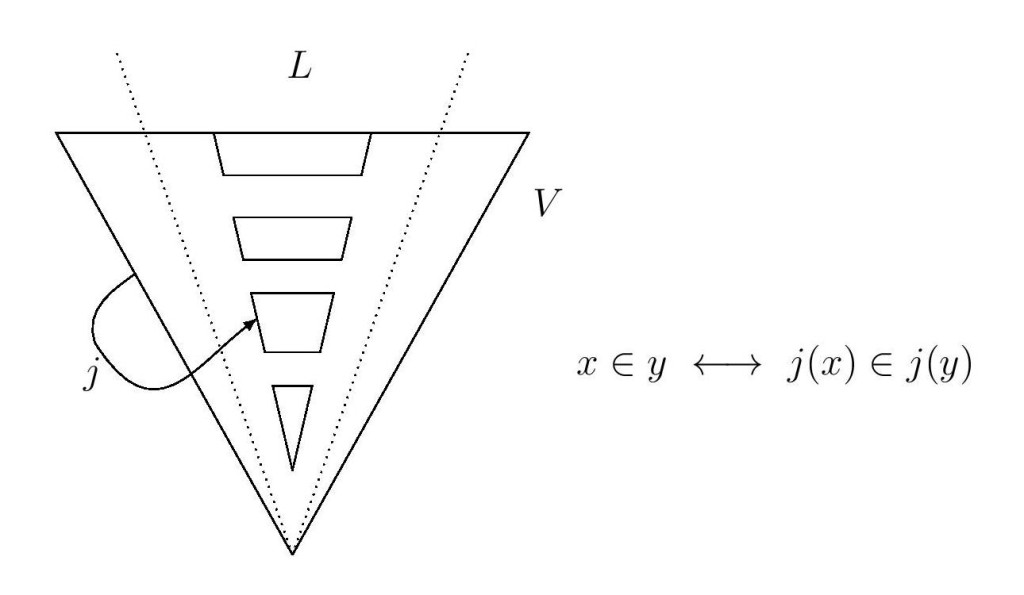
I shall describe the current state of knowledge concerning the question of whether there can be an embedding of the set-theoretic universe into the constructible universe.
Question.(Hamkins) Can there be an embedding
The notion of embedding here is merely that
Nevertheless, the question arises very naturally in the context of my previous work on the embeddability phenomenon, Every countable model of set theory embeds into its own constructible universe, where the title theorem is the following.
Theorem.(Hamkins) Every countable model of set theory
The methods of proof also established that the countable models of set theory are linearly pre-ordered by embeddability: given any two models, one of them embeds into the other; or equivalently, one of them is isomorphic to a submodel of the other. Indeed, one model
In the proof of that theorem, the embeddings
Currently, the question remains open, but we have some partial progress, and have settled it in a number of cases, including the following, on which I’ll speak:
- If there is an embedding
- If
- If
- If there is an embedding
- In the forcing extension
- If
- Every countable set
This is joint work of myself, W. Hugh Woodin, Menachem Magidor, with contributions also by David Aspero, Ralf Schindler and Yair Hayut.
See my related MathOverflow question: Can there be an embedding

Hi. All of this is very interesting stuff – of course, the notion of embedding (as you say) is very “non-elementary”. Still, I find intriguing the behavior of powers between V and L and the way forcing seems to block even these very weak embeddings. How crucial is the “countable” assumption in the theorem? Can you lift the linear ordering by embeddability to other cardinalities? If not, what would this be saying about countable models?
The countability assumption is crucial. In recent work, Gitman and Fuchs and I have provided
Dear Joel,
would you be so kind to keep in touch with me?
I am now professor emeritus of Tokyo Institute of Technology, and a researcher
at the Institute of Linguistics at Keio University.
Mostly I have been concerned with Lesniewski’s systems, esp. Ontology.
Sure, what would you like to discuss? You can also contact me by email jhamkins@gc.cuny.edu. I was in Japan at the Tokyo Institute of Technology in September: http://jdh.hamkins.org/universality-and-embeddability-ctfm-2015-japan/.