This will be a talk for the CUNY Logic Workshop at the CUNY Graduate Center, September 8, 2017, 2-3:30, room GC 6417.
Abstract. Consider the collection of all the models of arithmetic under the end-extension relation, which forms a potentialist system for arithmetic, a collection of possible arithmetic worlds or universe fragments, with a corresponding potentialist modal semantics. What are the modal validities? I shall prove that every model of arithmetic validates exactly S4 with respect to assertions in the language of arithmetic allowing parameters, but if one considers sentences only (no parameters), then some models can validate up to S5, thereby fulfilling the arithmetic maximality principle, which asserts for a model
The proof makes fundamental use of what I call the universal algorithm, a fascinating result due to W. Hugh Woodin, asserting that there is a computable algorithm that can in principle enumerate any desired finite sequence, if only it is undertaken in the right universe, and furthermore any given model of arithmetic can be end-extended so as to realize any desired additional behavior for that universal program. I shall give a simple proof of the universal algorithm theorem and explain how it can be used to determine the potentialist validities of a model of arithmetic. This is current joint work in progress with Victoria Gitman and Roman Kossak, and should be seen as an arithmetic analogue of my recent work on set-theoretic potentialism with Øystein Linnebo. The mathematical program is strongly motivated by philosophical ideas arising in the distinction between actual and potential infinity.

![King of Hearts / Wikimedia Commons / CC-BY-SA-3.0 [GFDL (http://www.gnu.org/copyleft/fdl.html) or CC BY-SA 3.0 (http://creativecommons.org/licenses/by-sa/3.0)], via Wikimedia Commons Empire_State_Building_New_York_March_2015](http://jdh.hamkins.org/wp-content/uploads/2017/08/Empire_State_Building_New_York_March_2015-188x300.jpg)

Dear Sir,
I may be off the mark, but historically isn’t the statement “Consider the collection of all the models…” precisely of the type that is rejected by those who only want to consider potential infinities ? I thought that they would not want to deal with as an actual object, but only talk about “
as an actual object, but only talk about “![[0;n]](https://s0.wp.com/latex.php?latex=%5B0%3Bn%5D&bg=ffffff&fg=000&s=0&c=20201002) for
for 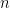 as large as one may whish” (a never-ending process rather than a given object).
as large as one may whish” (a never-ending process rather than a given object).
If so, then in what way is your work on “the distinction between actual and potential infinity”, rather than on “exploring the structure of all possible models” (possible, not potential) ?
Oh, yes, thank you for your comment. We discuss this issue at length in our paper (which is not yet available).
Indeed, although the mathematical project here is inspired by the issue of actualism versus potentialism, it is not meant to be taken as a piece of potentialist mathematics. The situation here is not unlike a logician who might use classical logic in order to analyze the power of a particular intuitionistic logical system.
My view of the project is that we seem able to use this kind of nonstandard analysis to provide a way in part to make sense of some views, such as ultrafinitism, which otherwise have had such a difficult time to be coherently expressed. What we have here in our potentialist system is a mathematical model that appears to share many of the main features that the ultrafinitists assert about the nature of mathematical reality, and which has a kind of friendly simulation of those views, if one adopts a nonstandard perspective, but of which we can provide a full mathematical account using the actualist mathematics at our disposal.
The conclusion is that our analysis seems to provide reasons to expect the ultrafinitist/potentialist perspective to have S4 as its potentialist validities, while opening the door to S5 for sentences.
In addition, our analysis tends to highlight some issues with philosophical significance, which we haven’t otherwise seen discussed enough. For example, using the arbitrary-extension model of potentialism rather than the end-extension model corresponds to the philosophical idea for an ultrafinitist that one might gain access to some large numbers, without necessarily yet having access to all smaller numbers. For example, perhaps it makes sense for an ultrafinitist to be able to analyze the number googol-plex-bang, which is a truly enormous number yet having a comparatively small description, without yet being committed to the actual existence yet of all the smaller numbers.
In our potentialist system, we nevertheless prove that still S4 is the class of validities for this version of potentialism.
Ultimately, my view of the philosophical value of the mathematical project is that the potentialist systems we are studying seem to exhibit many of the features that the potentialists seem to want, while avoiding the problematic issues, such as the commonly mentioned awkwardness for an ultrafinitist to assert the existence of a largest number. So we feel that by analyzing these potentialist systems, we gain philosophical insight into the potentialist viewpoint.
Thank you for the prompt reply. Looking forward to reading your paper then, and will try to digest it.