Lectures on Set Theory, Beijing, June 2025

Posted on by
4

This will be a lecture series at Peking University in Beijing in June 2025.

Announcement at Peking University

Course abstract. This will be a series of advanced lectures on set theory, treating diverse topics and particularly those illustrating how set theoretic ideas and conceptions shed light on core foundational matters in mathematics. We will study the pervasive independence phenomenon over the Zermelo-Fraenkel axioms of set theory, perhaps the central discovery of 20th century set theory, as revealed by the method of forcing, which we shall study in technical detail with numerous examples and applications, including iterated forcing. We shall look into all matters of the continuum hypothesis and the axiom of choice. We shall introduce the basic large cardinal axioms, those strong axioms of infinity, and investigate the interaction of forcing and large cardinals. We shall explore issues of definability and truth, revealing a surprisingly malleable nature by the method of forcing. Looking upward from a model of set theory to all its forcing extensions, we shall explore the generic multiverse of set theory, by which one views all the models of set theory as so many possible mathematical worlds, while seeking to establish exactly the modal validities of this conception. Looking downward in contrast transforms this subject to set-theoretic geology, by which one understands how a given set-theoretic universe might have arisen from its deeper grounds by forcing. We shall prove the ground-model definability theorem and the other fundamental results of set-theoretic geology. The lectures will assume for those participating a certain degree of familiarity with set-theoretic notions, including the basics of ZFC and forcing.

There will be ten lectures, each a generous 3 hours.

Lecture 1. Set Theory

This first lecture begins with fundamental notions, including the dramatic historical developments of set theory with Cantor, Frege, Russell, and Zermelo, and then the rise of the cumulative hierarchy and the iterative conception. The move to a first-order foundational theory. The Skolem paradox. The omission of urelements and the move to a pure set theory. We will establish the reflection phenomenon and the phenomenon of correctness cardinals, before providing some simple relative consistency results. We will compare the first-order approach to the various class theories and also lay out the spectrum of weak theories, including locally verifiable set theory, before discussing countabilism as an approach to set theory.

Lecture 2. Categoricity and the small large cardinals

We will discuss the central role and importance of categoricity in mathematics, highlighting this with results of Dedekind and Huntington, and with several examples of internal categorcity. Afterwards, we shall begin to introduce various small large cardinal notions—the inaccessible cardinals, the hyperinaccessibility hierarchy, Mahlo cardinals, worldly cardinals, other-worldly cardinals. We shall explain the connection with categoricity via Zermelo’s categoricity result. Going deeper, we discuss the possibility of categorical large cardinals and the enticing possibility of a fully categorical set theory.

Lecture 3. Forcing

We shall give an introduction to forcing, pursuing and comparing two approaches, via partial orders versus Boolean algebras. Forcing arises naturally from the iterative conception of the cumulative hierarchy, when undertaken in multi-valued logic. We shall see the principal introductory forcing examples, including the forcing to add a Cohen real, cardinal collapse forcing, forcing the failure of CH, forcing to add dominating reals, almost disjoint coding, iterated forcing, the forcing of Martin’s axiom, and the case of Suslin trees.

Lecture 4. Continuum Hypothesis

We tell the story of the continuum hypothesis, from Cantor’s initial conception and strategy, to Gödel’s proof of CH in the constructible universe, and ultimately Cohen’s forcing of ¬CH, establishing independence over ZFC. The CH is a forcing switch. We discuss the generalized continuum hypothesis GCH, and prove Easton’s theorem on the continuum function. Finally, we discuss various philosophical approaches to settling the CH problem, including Freiling’s axiom and the equivalence with ¬CH, and the role of the continuum hypothesis in providing a categorical theory of the hyperreals. Two equivalent formulations of CH in ZFC are not equivalent without AC.

Lecture 5. Axiom of Choice

We tell the story of the axiom of choice, beginning with a spectrum of equivalent formulations, including the linearity of cardinality. We discuss the abstract cardinal-assignment problem versus the cardinal-selection problem. We establish the truth of the axiom of choice in the constructible universe, as well as global choice, but ultimately the independence of the axiom of choice over ZF via forcing and the symmetric model construction method. Finally, we discuss the perfect predictor theorem and the box puzzle conundrum.

Lecture 6. Definability

We shall define and discuss the formal notion of definability in mathematics and set theory. Can every set be definable? We exhibit the phenomenon of pointwise definable models and their relevance for the Math Tea argument. We define the inner model HOD and explore its interaction with forcing, forcing V=HOD and also forcing V≠HOD. We reveal the coquettish nature of HOD, establishing the nonabsoluteness of HOD, showing furthermore that every model of set theory is the HOD of another model. We show how forcing generic filters can be definable in their forcing extensions. Finally, we shall exhibit a spectrum of paradoxical examples revealing various subtleties in the notion of definability.

Lecture 7. Truth

What is truth? We establish Tarski’s theorem on the nondefinability of truth, and establish the second incompleteness theorem via the Grelling-Nelson paradox. We analyze the connection between truth predicates and correctness cardinals. What is the consistency strength of having a truth predicate? Can a model of set theory contain its own theory as an element? Must it? We define the truth telling game. We shall force a definable truth predicate for HOD. We shall establish the nonabsoluteness of satisfaction.

Lecture 8. Forcing and large cardinals

Can large cardinals settle CH? Gödel had hoped so, but this is refuted by the Levy-Solovay theorem. We will prove forcing preservation theorems for large cardinals, and nonabsoluteness theorems. On the difference between lifting and extending measures. Laver indestructibility and the lottery preparation, via master condition arguments.

Lecture 9. Set-theoretic geology

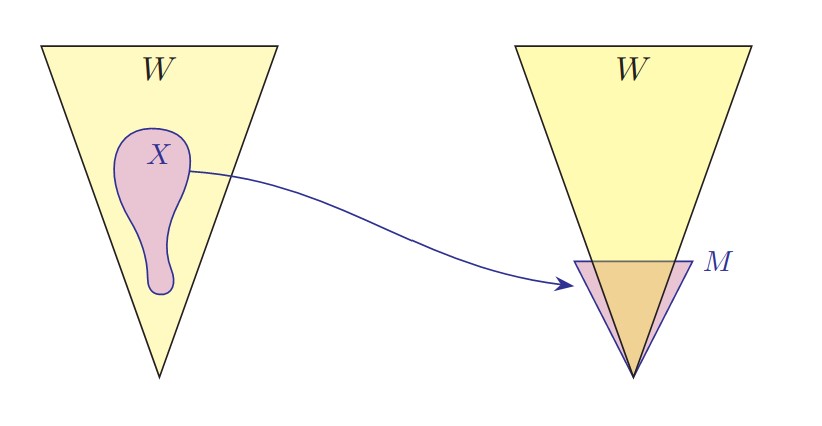
Looking down, we shall give an introduction to set-theoretic geology. We will prove the ground model definability theorem, using the cover and approximation properties. We shall define the Mantle and prove that every model of set theory is the Mantle of another model. We will discuss Bukovski’s theorem characterizing forcing extensions and prove Usuba’s theorems on the downward directedness of grounds.

Lecture 10. Set-theoretic potentialism

Looking up, we view forcing as a modality, viewing every model of set theory in the context of its generic multiverse. We shall investigate the modal logic of forcing with independent buttons and switches. We shall explore the other natural interpretations of set-theoretic potentialism and investigate their modal validities.

Comments or suggestions welcome.

Skolem’s paradox and the countable transitive submodel theorem, Leeds Set Theory Seminar, May 2025

Posted on by
2

This will be an online talk for the Leeds Set Theory Seminar, 21 May 2025 1pm BST. Contact the organizers (Hope Duncan) for Teams access.

Abstract: One can find in the philosophical research literature surrounding Skolem’s paradox a certain claim, referred to as the transitive submodel theorem, according to which every transitive model of set theory admits a countable transitive submodel of the same theory. Although the statement may initially appear plausible—perhaps one thinks it follows from an application of the downward Löwenheim-Skolem theorem—nevertheless it turns out that as a mathematical claim, it is overstated. There is no such theorem. In this talk I shall give a full account of the countable transitive submodel proposition, taken as a principle of set theory, showing from suitable hypotheses that counterexamples are possible and characterizing exactly the circumstances in which the principle does hold. Ultimately, the countable transitive submodel proposition should be seen as a certain anti-large cardinal principle that is equiconsistent with but independent of ZFC and refuted by all the moderately strong large cardinal notions. This is joint work in progress with Timothy Button, with thanks to W. Hugh Woodin.

Slides – Hamkins – On Skolem’s paradox – Leeds 2025Download

The Church of Logic podcast, April 2025

Posted on by
Reply

I was interviewed by Cody Roux for The Church of Logic podcast—a fascinating sweeping conversation on issues in the philosophy of mathematics and set theory, including what I described as a fundamental dichotomy between two perspectives on the nature of mathematics and what it is all about. Cody and I have affinities with opposite sides of this dichotomy, which made for a fruitful exchange.

A potentialist conception of ultrafinitism, Columbia University, April 2025

Posted on by
4


This will be a talk for the conference on Ultrafinitism: Physics, Mathematics, and Philosophy at Columbia University in New York, April 11-13, 2025.

Abstract. I shall argue in various respects that ultrafinitism is fruitfully understood from a potentialist perspective, an approach to the topic that enables certain formal treatments of ultrafinitist ideas, which otherwise often struggle to find satisfactory formalization.

Slides – Ultrafinitism – Columbia 2025 – HamkinsDownload

Handout format, without pauses: Slides – Ultrafinitism – Columbia 2025 – Hamkins – handout

The hierarchy of consistency strengths for membership in a computably enumerable set, Oxford Logic Seminar, May 2025

Posted on by
Reply

 This will be a talk for the Logic Seminar at the Mathematical Institute of the University of Oxford, 29 May 2025 5pm Andrew Wiles Building.

Abstract. For a given computably enumerable set W, consider the spectrum of assertions of the form n∈W. If W is c.e. but not computably decidable, it is easy to see that many of these statements will be independent of PA, for otherwise we could decide W by searching for proofs of n∉W. In this work, we investigate the possible hierarchies of consistency strengths that arise. For example, there is a c.e. set Q for which the consistency strengths of the assertions n∈Q are linearly ordered like the rational line. More generally, I shall prove that every computable preorder relation on the natural numbers is realized exactly as the hierarchy of consistency strength for the membership statements n∈W of some computably enumerable set W. After this, we shall consider the c.e. preorder relations. This is joint work with Atticus Stonestrom (Notre Dame).

Lecture notes – Consistency strength – Oxford – May 2025Download

Introduction to modal model theory, Panglobal Algebra and Logic Seminar, Boulder, March 2025

Posted on by
Reply

This will be a talk for the Panglobal Algebra and Logic seminar at the University of Colorado Boulder, March 12, 2025, 3:30pm MDT

The talk will be available live on Zoom. Contact the organizers for access.

Abstract. I shall introduce and describe the subject of modal model theory, in which one studies a mathematical structure within a class of similar structures under an extension concept, giving rise to mathematically natural notions of possibility and necessity, a form of mathematical potentialism. We study the class of all graphs, or all groups, all fields, all orders, or what have you; a natural case is the class of all models of a fixed first-order theory. In this talk, I shall describe some of the resulting elementary theory, particularly the remarkable expressive power of modal graph theory. This is joint work with my Oxford student Wojciech Wołoszyn.

Slides – Modal model theory – Hamkins – Boulder 2025Download

2025 William Reinhardt Memorial Lecture, Boulder

Posted on by
5

I am honored to be giving the 2025 William Reinhardt Memorial Lecture at the University of Colorado Boulder, March 11, 2025.

How we might have taken the Continuum Hypothesis as a fundamental axiom, necessary for mathematics

Abstract. I shall describe a simple historical thought experiment showing how our attitude toward the continuum hypothesis might easily have been very different than it is. If our mathematical history had been just a little different, I claim, if certain mathematical discoveries had been made in a slightly different order, then we would naturally have come to view the continuum hypothesis as a fundamental axiom of set theory, necessary for mathematics, indispensable even for the core ideas of calculus.

Hamkins_poster
Slides – CH could have been fundamental – Hamkins – Reinhardt Lecture 2025Download

On Skolem’s paradox and the transitive submodel theorem, Rust Belt Workshop in the Philosophy of Logic, Language, and Mathematics, February 2025

Posted on by
Reply

This will be a talk for the Rust Belt Workshop in the Philosophy of Logic, Language, and Mathematics, held at Ohio State University in Columbus, Ohio, February 8-9, 2025, University Hall (230 N Oval Mall, Columbus, OH) Room 386B.

Abstract. One can find in the philosophical research literature surrounding Skolem’s paradox a certain claim, referred to as the transitive submodel theorem, according to which every transitive model of set theory admits a countable transitive submodel of the same theory. Although the statement may initially appear quite plausible—perhaps one thinks it follows  from an application of the downward Löwenheim-Skolem theorem—nevertheless it turns out that as a mathematical claim, it is overstated, and there is no such theorem. It is a mistake, although an interesting mistake worth discussing. In this talk I shall give a full account of the countable transitive submodel proposition, taken as a principle of set theory, by showing from suitable hypotheses that counterexamples are possible, characterizing exactly the circumstances in which the principle does hold, and investigating the consistency strength of the proposition and also the consistency strength of its negation. Ultimately, the countable transitive submodel proposition should be seen as a certain anti-large cardinal principle that is equiconsistent with but independent of ZFC, refuted by all the moderately strong large cardinal notions.

This is joint work in progress with Timothy Button, with thanks to W. Hugh Woodin.

Slides – Hamkins – On Skolem’s paradox and the transitive submodel theorem – Rust Belt Workshop 2025Download

I will post a link to the paper when it is available.

The covering reflection principle – Oberwolfach January 2025

Posted on by
Reply

This was a talk I gave at the Set Theory Workshop at the Mathematisches Forschungsinstitut in Oberwolfach, Germany, 12-17 January 2025.

Abstract. The principle of covering reflection holds of a cardinal κ if for every structure B in a countable first-order language there is a structure A of size less than κ, such that B is covered by elementary images of A in B. Is there any such cardinal? Is the principle consistent? Does it have large cardinal strength? This is joint work with myself, Nai-Chung Hou, Andreas Lietz, and Farmer Schlutzenberg.

Slides – The covering reflection principle – Oberwolfach January 2025Download

The Human Podcast: 10 questions in 10 minutes

Posted on by
2

I had an enjoyable little discussion with Joe Murray of The Human Podcast, part of his new series, called 10 questions in 10 minutes, in which he asks his interview subjects for short answers to ten quick questions on their topic. Here is our conversation:

Joe was adamant about the 1 minute timeline for each question, and was holding up timers and giving me the 5 second warning and so forth, but of course, it was simply impossible! There was no way for me to contain my answers to the time limit.

Meanwhile, you can follow through to our previous, longer discussion here:

Every worldly cardinal admits a Gödel-Bernays structure

Posted on by
2

My Oxford student Emma Palmer and I have been thinking about worldly cardinals and Gödel-Bernays GBC set theory, and we recently came to a new realization.

Namely, what I realized is that every worldly cardinal κ admits a Gödel-Bernays structure, including the axiom of global choice. That is, if κ is worldly, then there is a family X of sets so that ⟨Vκ,∈,X⟩ is a model of Gödel-Bernays set theory GBC including global choice.

For background, it may be helpful to recall Zermelo’s famous 1930 quasi-categoricity result, showing that the inaccessible cardinals are precisely the cardinals κ for which Vκ is a model of second-order set theory ZFC2.

If one seeks only the first-order ZFC set theory in Vκ, however, then this is what it means to say that κ is a worldly cardinal, a strictly weaker notion. That is, κ is worldly if and only if Vκ⊨ZFC. Every inaccessible cardinal is worldly, by Zermelo’s result. But more, every inaccessible is a limit of worldly cardinals, and so there are many worldly cardinals that are not inaccessible. The least worldly cardinal, for example, has cofinality ω. Indeed, the next worldly cardinal above any ordinal has cofinality ω.

Meanwhile, to improve slightly on Zermelo, we can observe that if κ is inaccessible, then Vκ is a model of Kelley-Morse set theory when equipped with the full second-order complement of classes. That is, ⟨Vκ,∈,Vκ+1⟩ is a model of KM.

This is definitely not true when κ is merely worldly and not inaccessible, however, for in this case ⟨Vκ,∈,Vκ+1⟩ is never a model of KM nor even GBC when κ is singular. The reason is that the singularity of κ would be revealed by a short cofinal sequence, which would be available in the full power set Vκ)+1=P(Vκ), and this would violate replacement.

So the question is:

Question. If κ is worldly, then can we equip Vκ with a suitable family X of classes so that ⟨Vκ,∈,X⟩ is a model of GBC?

The answer is Yes!

What I claim is that for every worldly cardinal κ, there is a definably generic well order ⩽ of Vκ, so that the subsets definable in ⟨Vκ,∈,⩽⟩ make a model of GBC.

To see this, consider the class forcing notion P for adding a global well order ⩽, as Vκ sees it. Conditions are well orders of some Vα for some α<κ, ordered by end-extension, so that lower rank sets always preceed higher rank sets in the resulting order.

I shall prove that there is a well-order ⩽ that is generic with respect to dense sets definable in ⟨V,∈⟩.

For this, let us consider first the case where the worldly cardinal κ has countable cofinality. In this case, we can find an increasing sequence κn cofinal in κ, such that each κn is Σn-correct in Vκ, meaning Vκn≺ΣnVκ.

In this case, we can build a definably generic filter G for P in a sequence of stages. At stage n, we can find a well order up to κn that meets all Σn definable dense classes using parameters less than Vκn. The reason is that for any such definable dense set, we can meet it below κn using the Σn-correctness of κn, and so by considering various parameters in turn, we can altogether handle all parameters below Vκn using Σn definitions. That is, the nth stage is itself an iteration of length κn, but it will meet all Σn definable dense sets using parameters in Vκn.

Next, we observe that the ultimate well-order of Vκ that arises from this construction after all stages is fully definably generic, since any definition with arbitrary parameters in Vκ is a Σn definition with parameters in Vκn for some large enough n, and so we get a definably generic well order ⩽. Therefore, the usual forcing argument shows that we get GBC in the resulting model ⟨Vκ,∈,Def(Vκ)⟩, as desired.

The remaining case occurs when kappa has uncountable cofinality. In this case, there is a club set C⊆κ of ordinals γ∈C with Vγ≺Vκ. (We can just intersect the clubs Cn of the Σn-correct cardinals.) Now, we build a well-order of Vκ that is definably generic for every Vγ for γ∈C. At limits, this is free, since every definable dense set in V_lambda with parameters below is also definable in some earlier Vγ. So it just reduces to the successor case, which we can get by the arguments above (or by induction). The next correct cardinal γ above any ordinal has countable cofinality, since if one considers the next Σ1-correct cardinal, the next Σ2-correct cardinal, and so on, the limit will be fully correct and cofinality ω.

The conclusion is that every worldly cardinal κ admits a definably generic global well-order on Vκ and therefore also admits a Gödel-Bernays GBC set theory structure ⟨Vκ,∈,X⟩, including the axiom of global choice.

The argument relativizes to any particular amenable class A⊆Vκ. Namely, if ⟨Vκ,∈,A⟩ is a model of ZFC(A), then there is a definably generic well order ⩽ of Vκ such that ⟨Vκ,∈,A,⩽⟩ is a model of ZFC(A,⩽), and so by taking the classes definable from A and ⩽, we get a GBC structure X including both A and ⩽.

This latter observation will be put to good use in connection with Emma’s work on the Tarski’s revenge axiom, in regard to finding the optimal consistency strength for one of the principles.

The computable surreal numbers, Notre Dame Logic Seminar, December 2024

Posted on by
Reply

This will be a talk for the Notre Dame Logic Seminar, 3 December 2024, 2:00pm, 125 Hayes-Healey.

Abstract. I shall give an account of the theory of computable surreal numbers, proving that these form a real-closed field. Which real numbers arise as computable surreal numbers? You may be surprised to learn that some noncomputable real numbers have computable surreal presentations, and indeed the computable surreal real numbers are exactly the hyperarithmetic reals. More generally, the computable surreal numbers are exactly those with a hyperarithmetic surreal sign sequence. This is joint work with Dan Turetsky, but we subsequently found that it is a rediscovery of earlier work of Jacob Lurie.

Lecture notes:

Lecture Notes – Computable surreal numbers ND Dec 2024Download

See related MathOverflow posts:

Also see my elementary introduction to the surreal numbers: The surreal numbers

Determinateness of truth does not come for free from determinateness of objects, Singapore, November 2024

Posted on by
Reply

 This will be a talk for the (In)determinacy in Mathematics conference at the National University of Singapore, 20-22 November 2024

Abstract. I shall discuss the question whether we may regard determinateness of truth as flowing from determinateness of objects in a mathematical structure. I shall showcase several results in the model theory of set theory and arithmetic that seem to speak against this. For example, there are two models of ZFC set theory that share exactly the same arithmetic structure of the natural numbers ⟨ℕ,+,·,0,1,<⟩, what they each view as the standard model of arithmetic, but they disagree about which arithmetic sentences are true in that structure. There are models of ZFC set theory with the same arithmetic structure and the same arithmetic truth, but which disagree on truth-about-truth, or that agree on that, but disagree on higher levels of iterated truth, at any desired level. There are models of set theory with the same natural numbers and real numbers, but which disagree on projective truth. There are models of ZFC that have a rank initial segment Vθ in common, but they disagree about whether it is a model of ZFC. All these examples show senses in which determinateness about objects does not seem to cause determinateness about truth. (This is joint work with Ruizhi Yang.)

Slides – Determinateness of truth – Singapore 2024Download

The covering reflection theorem, Madison Logic Seminar, October 2024

Posted on by
2

This will be a talk at the UW Madison Logic Seminar on 22 October 2024.

Abstract. The principle of covering reflection holds of a cardinal κ if for every structure B in a countable first-order language there is a structure A of size less than κ, such that B is covered by elementary images of A in B. Is there any such cardinal? Is the principle consistent? Does it have large cardinal strength? This is joint work with myself, Nai-Chung Hou, Andreas Lietz, and Farmer Schlutzenberg.

The talk will reportedly streamed online, so kindly contact the organizers for access.

I will be staying in Madison for a few days to talk logic with researchers there.

Slides – The covering reflection principle – Madison Oct 2024Download

Video Player

Infinite-time computable analogues of the universal algorithm, Generalized Computability Theory Workshop, Spain, August 2024

Posted on by
2

This will be a talk at the Generalized Computability Theory workshop in Castro Urdiales, Spain, a beautiful setting on the sea near Bilbao, 19-23 August 2024.

Abstract. I shall present infinite-time computable analogues of the universal algorithm, which can in principle produce any desired output stream, if only it is run in the right set-theoretic universe, and then extended as desired in further universes.

Slides – Infinitary universal algorithm – Spain 2024 – HamkinsDownload